Finding math at home

If you’re bored at home, and in a mathematical mood, you might go online and start reading about some interesting math (yes, you might!). But there are other options: you could just explore your own home, and try to find some math that might be hiding somewhere! Actually, there is math lurking all over your house, if you just have the inspiration to experiment and play. Make up your own questions, and see what happens!

For instance, if you were in your kitchen and noticed seven cups in the dishwasher waiting to be put away, you might take them out and place them on the table in a row, all face up:

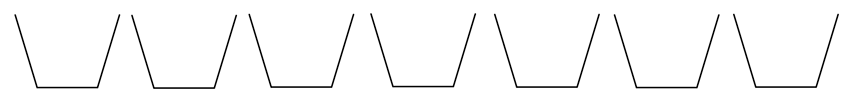
And since your favorite number is 4, you might wonder what your row of glasses would look like if you turned four glasses upside down, say like so:

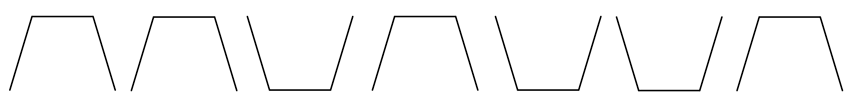
And what if you then turned another 4 glasses upside down? Any four, including some of the ones you just turned?

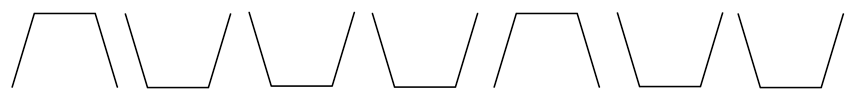
And you kept doing that?

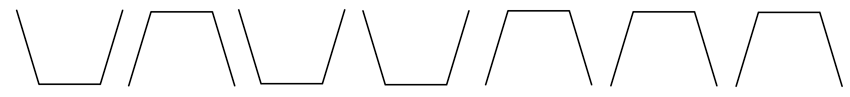
After you do this for a while, you might start wondering about all the different configurations of glasses you could actually get by performing these steps. What kind of questions could you ask about the results of this process? Get seven glasses, and go through some of these steps yourself! Experiment and wonder!

A very natural question

We started with all the glasses face up. And if you reversed your steps at any moment, you could get back to that initial configuration, all glasses face up. But… could you ever get all the glasses face down? Hmm… that’s kinda interesting. Did you ever see a row of face down glasses when you tried this? If you only ever turned over 4 glasses at a time, we’re betting that you didn’t get to see that, and that’s because it’s not possible to do!

As it turns out, if you start with 7 face up glasses, turn over any 4 of them at a time for as many steps as you like, you will never be able to have all the glasses face down. Of course, now the question is… why is this? How can we be sure that there’s no way to get them all face down? Maybe we just missed the crucial step somewhere? We need an explanation!

Thinking about parity for the big picture

The key to explaining why we can’t get all cups face down is to think about parity, which is the evenness or oddness of a number. We start with 7 face up glasses; 7 is odd. We need to end with 0 face up glasses; 0 is even (why?). Now, the numbers are important, but let’s get the bigger picture here by focusing not on the quantities 7 and 0, but on their parity. What we want at some point in our process is for the parity of the number of face up glasses to change from odd (7) to even (0). But in fact, as you can check in our pictures above, and in your own experiment, the parity of the number of face up glasses is always the same: odd! The number of face up glasses changes, but there are always an odd number of them, and that’s ensures that we’ll never reduce their number to 0, an even number. Feel free to check these details for yourself!

Keep experimenting!

Once you realize what’s going on with 7 glasses, try 8 glasses… you’ll find that you can solve the puzzle then! What about 9 glasses? 10? What patterns can you find? What if you change the number of glasses you’re allowed to flip? To 3 instead of 4? Gather up your glasses, experiment, make charts and tables, and find out all sorts of interesting conclusions for yourself! One of the true joys of math isn’t simply reading what others have to say, but finding out what the math has to say for itself, and what you have to say about it! Stuck on something? Get in touch with us!

Find this post interesting? Follow the blog using the link at the top of the page to get notified when new posts appear!

Want awesome tips and a mini-challenge, all designed to help you build vital problem-solving and critical thinking skills in your child? Click here to sign up for our monthly newsletter!